Low-energy electron diffraction (LEED) is based on the diffraction of electrons by the Bragg planes of a single-crystalline sample. Due to the electrons’ low energy (typically 10–200 eV), their mean free path in the material is limited to the first few atomic layers, and so LEED gives information only on the surface’s topological structure.
The diffraction of electrons by a crystal’s lattice was first observed experimentally by Davisson and Germer in 1927 [53]. The experiment was originally intended to study the surface of a nickel target, with the hypothesis that even the smoothest surface would be too rough for specular reflection, and would instead diffuse an incident electron beam. However, after the accidental oxidation of the target, the sample was cleaned in a high-temperature oven, thus creating single crystallites on the surface. When the experiment was repeated, they observed that the electrons were scattered as if by a diffraction grating, and using Bragg’s law it was shown that the electrons were diffracting from the nickel crystal planes. This serendipitous result bears mentioning because not only is it the first experimental evidence of the wave–particle duality of matter, but was also important for the development of quantum mechanics and the Schrödinger equation.
The diffraction of X-rays from crystalline solids was explained by William Lawrence Bragg by describing the solid as a series of parallel planes, and the characteristic spots observed originating from points of constructive interference of the X-rays. A similar treatment can be performed for electron diffraction, since the electrons’ de Broglie wavelength is comparable to the interatomic spacing, however an alternate theory is described in Section 2.2.3 for scattering due to a surface.
As shown in Figure 2.4, depending on the angle of diffraction from the atoms in the crystal, the path length difference between the scattered waves reflecting from different layers of the crystal can cause them to interfere constructively (Figure 2.4a) or destructively (Figure 2.4b).
The path difference is given by d sin(θ), where d is the interlayer spacing, and θ is the angle subtended between the incident and diffracted waves. In the case of Figure 2.4a, the path length difference between the two reflected waves is an integer number of wavelengths (2λ). This is equivalent to a phase shift of 2π, and so the two waves constructively interfere. In contrast, in Figure 2.4b the path difference is equal to one and a half wavelengths, i.e. a phase shift of π, leading to destructive interference.
The relation derived by Bragg for constructive interference between two waves at normal incidence was:
 | (2.1) |
where n is an integer and λ is the wavelength of the incident wave.
It is clear from Equation 2.1 that some angles will lead to maxima in the diffracted beam intensity, but it should be noted that in the case of the diagram, if only two atoms are involved, there will be a smooth transition between maxima and minima. In practice, the size of the electron beam in LEED means that many atoms diffract the beam, mainly sharp peaks are observed, surrounded by areas of destructive interference. For this reason LEED is an averaging technique.
Bragg diffraction (and hence LEED) does not directly measure real-space atomic distances. Instead, the distance between diffraction spots is inversely proportional to the interatomic spacing, d, and the nλ term is related to the number of wavelengths that fit between atoms. Therefore, a LEED pattern describes reciprocal space.
Reciprocal space effectively represents the periodicity of a Bravais lattice. This is equivalent to performing a Fourier transform on a periodic function.
Consider a vector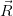 
, which represents the Bravais lattice, and a plane wave
ei
⋅
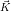= cos(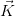
⋅
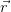) + i sin(
⋅
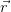) which has the same periodicity as the Bravais
lattice.
If their periodicities are equal, then for any point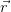 
:
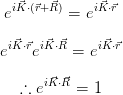
The set of vectors 
which satisfy the above relation describes the reciprocal
lattice. It is also noted that the reciprocal lattice is itself a Bravais lattice,
and the reciprocal of the reciprocal lattice returns the original real-space
lattice.
For an infinite 3-dimensional lattice, defined by its primitive vectors (
,
,
),
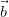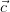the reciprocal lattice (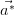
,
,
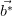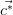) is defined as:
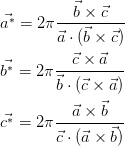 | (2.2) (2.3) (2.4) |
The de Broglie wavelength of an electron, λe is given by:
![∘ -------
---h---- -1.5---
λ = √2meE---; λe[nm ] ≈ Ee[eV]](thesis37x.png) | (2.5) |
where me and Ee are the electron’s mass and kinetic energy, respectively.
Consider a beam of electrons impinging on a 3-dimensional crystal, as in
Section 2.2.1. For an incident electron with wave vector 
= 2π∕hλ0, and a
scattered wave vector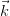 
= 2π∕hλ, the von Laue condition for constructive
interference states that:
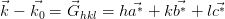 | (2.6) |
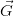hkl is a vector
of the reciprocal lattice.∗
Only elastic scattering is considered, and so energy is conserved, i.e. |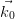
| = |
|.
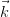As mentioned, the mean free path of electrons within a crystal is small and so
only the first few atomic layers play a role in the diffraction. Therefore, there are
no diffraction elements perpendicular to the surface, and the lattice can be
considered as a 2-dimensional series of rods extending from the surface lattice
points.
Following from this, the 2-dimensional simplification of Equation 2.6 and the reciprocal lattice vectors become
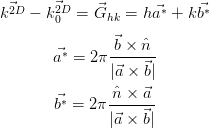 | (2.7) (2.8) (2.9) |
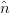is the surface normal unit vector.
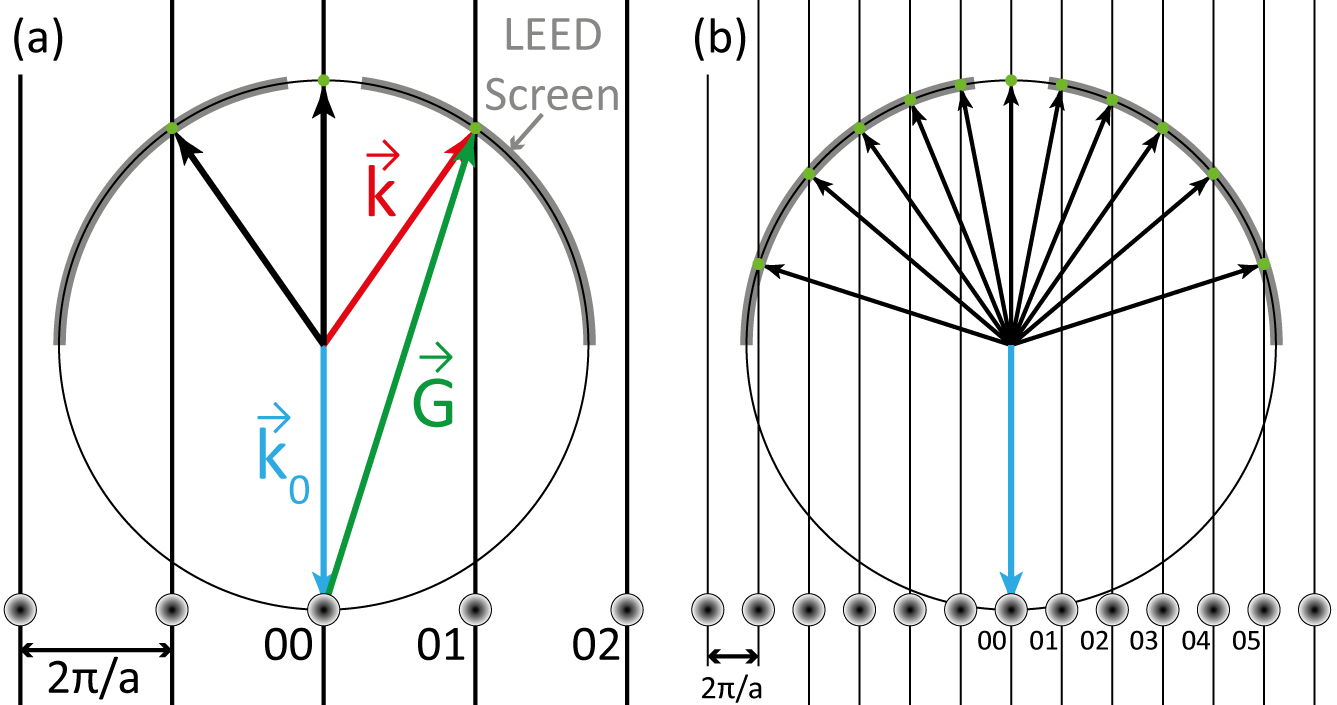
|, and
because LEED is performed using a beam of electrons impinging normal to
the surface, by default the incident wave vector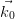 
lies parallel to the vertical
00 rod. The spots (rods) are numbered by their hk value. Points where the
rods cross the sphere coincide with the Laue condition (Equation 2.7). (a)
Spots are then formed on the fluorescent LEED screen at these points of
constructive interference. (b) at higher kinetic energies, the Ewald sphere
radius increases and more rods cross the sphere, thus more LEED spots
(green circles) are visible.
A useful visualisation of the effect of Equation 2.7 is the Ewald sphere, shown in Figure 2.5.
The Ewald sphere illustrates the points of constructive interference formed by the incident and diffracted electron waves. The upper half of the sphere can be considered as the hemispherical fluorescent screen of the LEED apparatus, and from Figure 2.5b it is clear how a higher kinetic energy leads to more LEED spots visible on the screen.
The set-up for LEED is shown in Figure 2.6a.
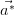and 
are shown in white.
Electrons are emitted from the electron gun by an accelerating voltage V , and are diffracted by the sample. They then pass through a series of up to four grids before colliding with a fluorescent coating on the screen, where light is emitted. The first and last grids are held at the ground potential to confine the field. The central grids are kept close to V at V ± δV , in order to select only elastically scattered electrons. Finally, the electrons are post-accelerated by a high voltage placed on the screen, in order to maximise the efficiency of the fluorescence.
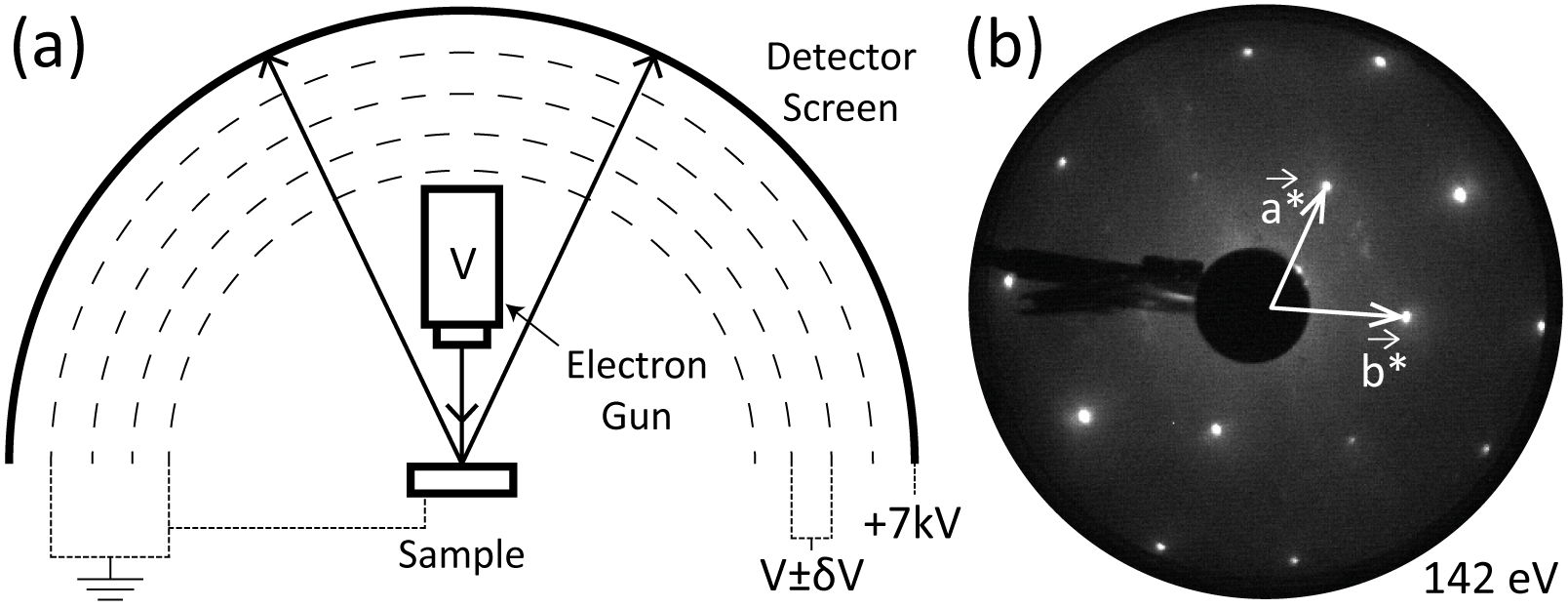
A LEED pattern obtained from the clean Mo(110) surface is shown in Figure 2.6b, showing the hexagonal reciprocal lattice representative of the close-packed (110) surface of a body-centred cubic crystal.