Sponsored by

Boston College
HMI
|
The 3rd William
Rowan
Hamilton Geometry and
Topology Workshop
September 6-8, 2007
The Hamilton Mathematics
Institute,
Trinity College Dublin
Pro-p
groups and low dimensional topology
Schedule of Talks
Time
|
Speaker
|
Talk
|
Thursday
|
|
9.00
- 10.00
|
Marc
Lackenby
|
Links
between low-dimensional topology and profinite group theory |
10:15
- 11:15
|
Alexander
Lubotzky
|
Property
tau and Hyperbolic Manifolds
|
11:15
- 11:45
|
DISCUSSION |
11:45
- 1:30
|
LUNCH |
1:30
- 2:30
|
Andrzej
Zuk
|
Automata Groups |
2:45
- 3:45
|
Peter
Shalen |
4-free
groups and geometry of hyperbolic manifolds |
4:00
- 5:00
|
Martin
Kassabov
|
Finite
simple groups and expander graphs
|
Friday
|
|
9:00
- 10:00
|
Dan
Segal
|
Words
|
| 10:15
- 11:15 |
Alan
Reid
|
Heegaard
genus and Property tau for hyperbolic 3-manifolds |
11:15
- 11:45
|
DISCUSSION |
| 11:45
- 1:30 |
LUNCH |
| 1:30
- 2:30 |
Tim
Cochran
|
Homology
and p-series of Groups
|
| 2:45
- 3:45 |
Andrei
Jaikin-Zapirain
|
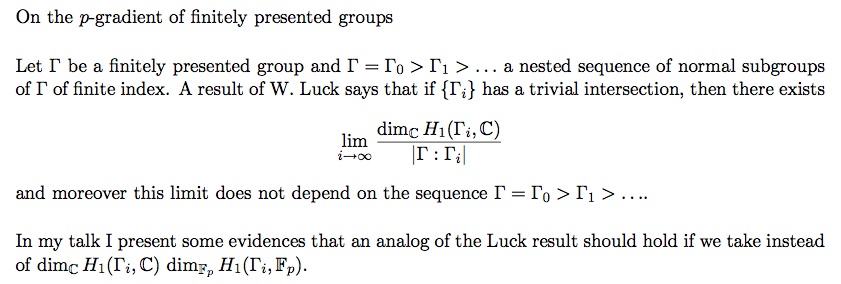
On
the p-gradient of finitely presented groups
|
| 4:00
- 5:00 |
Shelly
Harvey
|
Iterated
torsion-free abelian covers and L^2-Betti numbers of
3-manifolds
|
Saturday
|
|
| 9:00
- 10:00 |
Laurent
Bartholdi
|
Amenability
of Groups and Algebras |
| 10:15
- 11:15 |
Nigel
Boston |
Random
Pro-p Groups and Random Galois Groups |
11.15
- 12.15
|
DISCUSSION |
| END OF
WORKSHOP |
Format
The
three day directed workshop
will consist of background talks in the
morning, followed by a question-and-answer session, and then afternoon
lectures which will describe current
research.
The first two
days of
the
workshop there will be two morning talks and three afternoon talks. On
the last day, there will be two morning talks followed by a
discussion session in which participants
will be encouraged to describe parallels that they observed between the problems and techniques used in the various fields. A list of
observed parallels, open problems, and
likely future research directions will
be compiled for general dissemination.
The HMI will
publish the
workshop lectures and results as a series of notes. The notes will be
disseminated in paper form to other
institutes as well as be posted
on
the HMI website.
|
Abstracts
Marc Lackenby (Oxford University)
Links between low-dimensional topology and profinite group theory
Abstract: I'll give a survey of some of the known links between these
two areas. On the one hand, most of the key open questions in
3-manifold theory are concerned with finite-sheeted covering spaces,
and so profinite group theory has obvious applications here. On the
other hand, topological and geometric methods seem to be able to
establish facts about the finite index subgroups of finitely presented
groups that are not obvious algebraically. I'll also suggest some
possible areas of future research, particularly in relation to groups
with fast subgroup growth.
Alexander Lubotzky (Hebrew University)
Property tau and Hyperbolic Manifolds
We will give a short introduction to Property T, property tau and
expanders. Then we will show how these concepts were used to solve some
cases of the 'virtual first Betty number conjecture' for hyperbolic
manifolds. We will also give some introduction to the recent idea of
Lackenby how to use these concepts to try to prove the virtual Haken
conjecture for hyperbolic 3-manifolds. If time permits we will connect
it also with pro-p groups.
Andrzej Zuk (Université Paris VII)
Automata Groups
Peter Shalen (University of Illinois, Chicago)
4-free groups and geometry of hyperbolic manifolds
This is joint work with Marc Culler.
A group Gamma is said to be k-free,
for a given positive integer k, if
every subgroup of Gamma with rank at most k is free. We prove that if M
is a closed, orientable hyperbolic 3-manifold such that pi_1(M) is
4-free, then M contains a point P which is (log 7)-semithick. This
means that there is a cyclic subgroup Z of pi_1(M,P) such that every
homotopically non-trivial loop based at P which has length < log 7
represents an element of Z.
I will discuss the proof of this result and some applications to the
study of hyperbolic volume. The proof involves a deep argument about
the nerve of a covering of hyperbolic 3-space by displacement
cylinders, and uses a recent group-theoretic result, recently proved by
Kent and independently by Louder-McReynolds, that if two rank-2
subgroups of a free group have a proof, using Louder's folding
machinery on graphs of spaces, have rank--2 intersection then they have
rank-2 join. The applications to volume involve finding a (log
7)-semithick point of M and a (log 3)-thick point of M which are
separated by a suitably large distance. Both the proof of the theorem
and the new arguments in the applications make strong use of the
generalized log(2k-1) theorem.
Martin Kassabov (Cornell University)
Finite simple groups and expander graphs
joint work with A.Lubotzky and N. Nikolov
A finite graphs with large spectral gap are called expanders. These
graphs have many nice properties and have many applications. It is easy
to see that a random graph is an expander but constructing an explicit
examples is very difficult. All known explicit constructions are based
on the group theory --- if an infinite group G has property T (or its
variants) then the Cayley graphs of its finite quotients form an
expander family.
This leads to the following question: For which infinite families of
groups G_i, it is possible to find generating sets S_i which makes the
Cayley graphs expanders?
The answer of the question is known only in few cases. It seems that if
G_i are far enough from being abelian then the answer is YES. However
if one takes `standard' generating sets the resulting Cayley graphs are
not expanders (in many cases).
I will describe a recent construction which answers the above
question in the case of the family of almost all finite simple groups.
If S is a FSG it is possible to construct explicit generating sets F_S,
such that the Cayley graphs C(S,F_S) are expanders, and the expanding
constant can be estimated.
Dan Segal (Oxford University)
Words
A survey of recent and older results about verbal width of
groups, with an emphasis on profinite groups.
Alan Reid (University of Texas, Austin)
Heegaard genus and Property tau for hyperbolic 3-manifolds
We will discuss the proof and applications of the following result:
Every finitely generated Kleinian group has a cofinal tower of finite
index normal subgroups that has Property tau.
Tim Cochran (Rice University)
Homology and p-series of Groups
We discuss conditions under which a group homomorphism induces
isomorphisms (respectively) on quotients of the group by the terms
of the lower central p-series, the derived p-series, or on the
pro-p completions. These homological conditions generalize
Stallings' theorem that a Z_p homology equivalence induces an
isomorphism on the pro-p completions. We give some applications
to 3-manifolds. We give examples of hyperbolic rational homology
3-spheres with the same pro-p completion as a 3-manifold with
nilpotent fundamental group. We give examples of hyperbolic
rational homology 3-spheres that have the same pro-p completion
as Z_p*Z_p...*Z_p, but admit no map onto this free product.
Andrei Jaikin Zapirain (Universidad
Autónoma,
Madrid)
Shelly Harvey (Rice University)
Iterated torsion-free abelian covers and L^2-Betti numbers of
3-manifolds
We will investigate L^2-Betti numbers associated to certain
iterated torsion-free abelian covers of 3-manifolds. In the case that
the group of the cover is residually finite, these can be interpreted
as the (weighted) limit of the betti numbers of some finite covers. We
will show that the L^2-Betti numbers satisfy a certain monotonicity
relation. We will also give some examples of 3-manifolds
where the first Betti numbers cannot grow linearly in certain types of
finite covers.
Laurent Bartholdi (Ecole Polytechnique Federale
Lausanne)
Amenability of Groups and Algebras
The amenable/nonamenable dichotomy is an important tool in Group
Theory, in part because of its various algebraic, geometric and
analytic interpretations. Another important invariant is "word growth";
if a group has subexponential growth then it is amenable, while the
converse does not hold -- but see below!
I will define a natural notion of amenability for associative algebras
(first considered by Gromov), and explain its relation to amenability
of groups.
I will then prove the following result: let $G$ be a group, and let
$(\gamma_n)$ be its lower central series. If $G$ is amenable, then the
rank of $\gamma_n/\gamma_{n+1}$ increases subexponentially in $n$. This
answers a question by Vershik; the proof resides in relating
amenability to growth in an appropriate algebra.
As a corollary, all the groups constructed by Golod and Shafarevich
groups are non-amenable. This gives the first examples ofnon-amenable,
residually-finite torsion groups.
Nigel Boston (University of Wisconsin, Madison)
Random Pro-p Groups and Random Galois Groups
Dunfield and Thurston studied how the distribution of
finite quotients of a random g-generator g-relator abstract
group compares with that of the fundamental group of a random
3-manifold obtained from a genus-g Heegard splitting. We
consider analogous questions for random g-generator g-relator pro-p
groups and for Galois groups of maximal pro-p extensions unramified
away from a finite set S of primes with |S| = g.
|
|

